
Now, unlike an oscillator, Moving Averages are not restricted to a number within a band or a set range of numbers.

Moving Averages takes a set of data (closing prices over a specified time period) and outputs their average price. EMA = x multiplier + EMA(previous day) Double Exponential Moving Average Calculation Double EMA = 2*EMA – EMA (EMA) Triple Exponential Moving Average Calculation Triple EMA = (3*EMA – 3*EMA(EMA)) + EMA(EMA(EMA)) The basics Calculate the Multiplier (2 / (Number of Periods + 1) therefore (2 / (5+1) = 33.333%įor the first EMA, we use the SMA(previous day) instead of EMA(previous day). Calculate the SMA (Period Values / Number of Periods)Ģ. There are three steps to calculate the EMA.
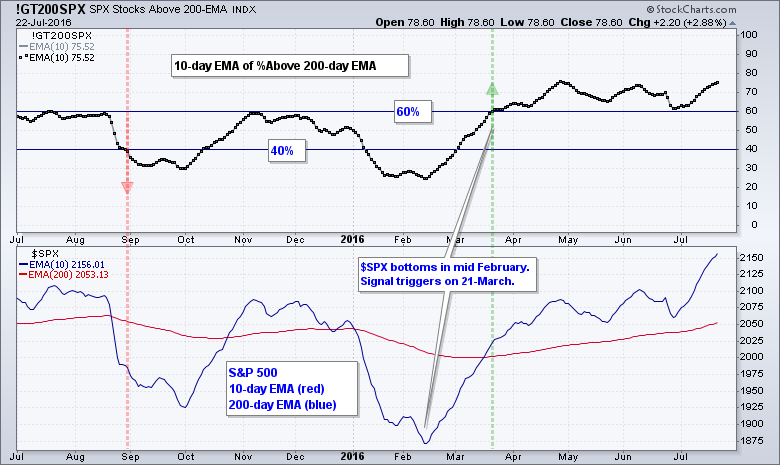
200 SMA VS 200 EMA SERIES
To clarify, old data points retain a multiplier (albeit declining to almost nothing) even if they are outside of the selected data series length. The major difference with the EMA is that old data points never leave the average. WMA 115 / 15 = 7.6667 Exponential Moving Average (EMA)Įxponential Moving Average is very similar to (and is a type of) WMA. WMA = (Sum of Weighted Averages) / (Sum of Weight) An example of a 5 period WMA Sum of Period Values / Number of Periods The most recent period has the most weight). Is similar to the SMA except it adds a weight (multiplier) to each period.
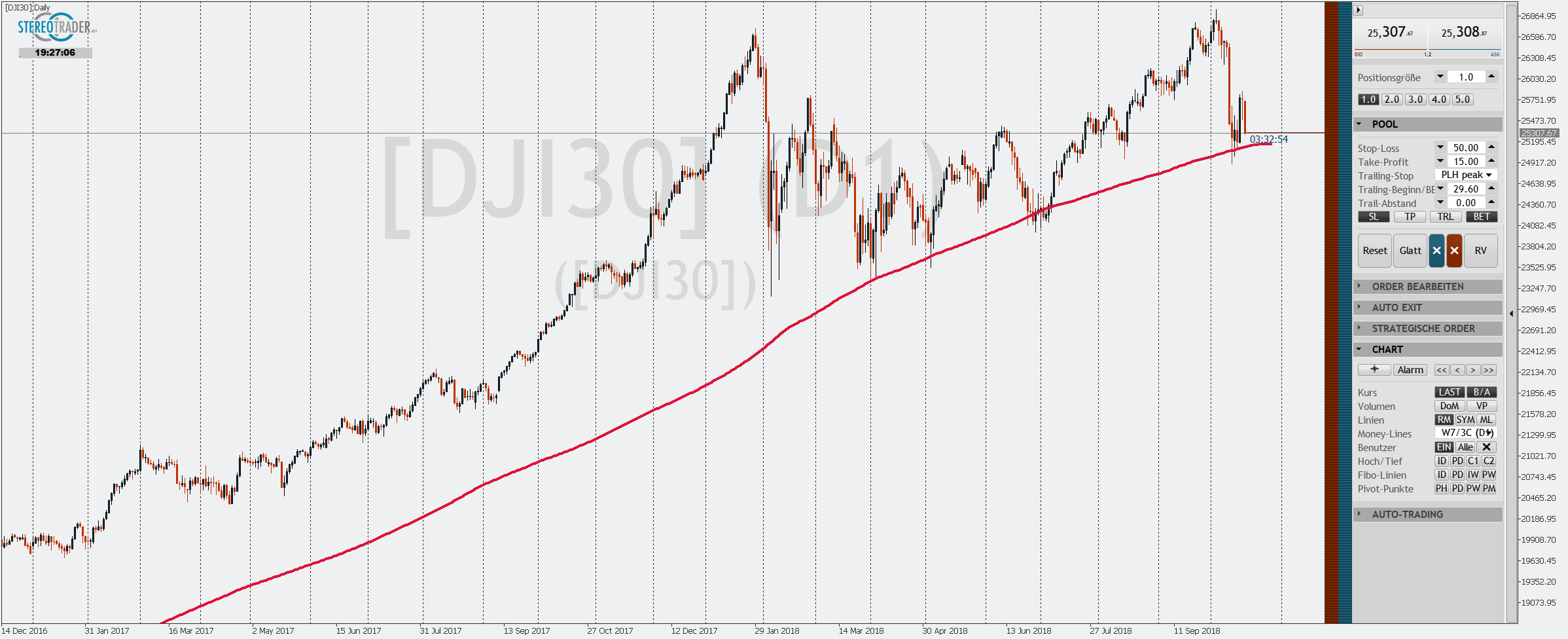
Then, just like the SMA, once a new data point is added to the beginning, the oldest data point is thrown out. Each point within the period is assigned a multiplier (largest multiplier for the newest data point and then descends in order) which changes the weight or significance of that particular data point. Weighted Moving Average is similar to the SMA, except the WMA adds significance to more recent data points. Third Day of 3 Period SMA: (7 + 8 + 9) /3 = 8 Weighted Moving Average (WMA) Second Day of 3 Period SMA: (6 + 7 + 8) / 3 = 7 CALCULATIONAn example of a 3 period SMA Sum of Period Values / Number of Periodsįirst Day of 3 Period SMA: (5 + 6 + 7) / 3 = 6 As each new day ends, the oldest data point is dropped and the newest one is added to the beginning. This means that each day in the data set has equal importance and is weighted equally. Simple Moving Average is an unweighted Moving Average. They typically differ in the way that different data points are weighted or given significance. However, there are a few different types of moving averages. Moving Averages visualize the average price of a financial instrument over a specified period of time. Most notable are the Simple Moving Average (SMA), the Exponential Moving Average (EMA) and the Weighted Moving Average (WMA). There are a few different types of Moving Averages which all take the same basic premise and add a variation. In fact, Moving Averages form the basis of several other well-known technical analysis tools such as Bollinger Bands and the MACD. Because a Moving Average is a lagging indicator and reacts to events that have already happened, it is not used as a predictive indicator but rather an interpretive one, used for confirmations and analysis. Noise is made up of fluctuations of both price and volume. Essentially, Moving Averages smooth out the “noise” when trying to interpret charts. A Moving Average is a good way to gauge momentum as well as to confirm trends, and define areas of support and resistance. Moving Average (MA) is a price based, lagging (or reactive) indicator that displays the average price of a security over a set period of time.


 0 kommentar(er)
0 kommentar(er)
